Odd-frequency Superconductivity

In general, the symmetry of a superconductor can be characterized by studying the anomalous Green’s function:

$F_{1;2}=-i\langle T \psi_{1}\psi_{2}\rangle$

where $\psi_i$ annihilates an electron with indices labeling spin, $\sigma_i$ , position, $\textbf{r}_i$ , time, $t_i$ , and orbital/band degrees of freedom, $\alpha_i$ , and $T$ is the time-ordering operator. Using the fermionic properties of electrons it is straightforward to show that: $F_{1;2}=-F_{2;1}$ . This relation tells us that the wavefunction describing the Cooper pairs, $\Psi$ , must obey $\mathcal{S}\mathcal{P}\mathcal{O}\mathcal{T}\Psi=-\Psi$ where: $\mathcal{S}$ acts on spin ( $\sigma_1\leftrightarrow\sigma_2$ ); $\mathcal{P}$ is the spatial parity operator ( $\textbf{r}_1\leftrightarrow\textbf{r}_2$ ); $\mathcal{O}$ interchanges orbital degrees of freedom ( $\alpha_1\leftrightarrow\alpha_2$ ); and $\mathcal{T}$ reverses the time coordinates ( $t_1\leftrightarrow t_2$ ). Using this property of $\Psi$ together with the fact that all four transformations square to the identity, the possible symmetries of the Cooper pair wavefunction may be divided into 8 different classes based on how they transform under $\mathcal{S}$ , $\mathcal{P}$ , $\mathcal{O}$ , and $\mathcal{T}$ :

Rendered by QuickLaTeX.com

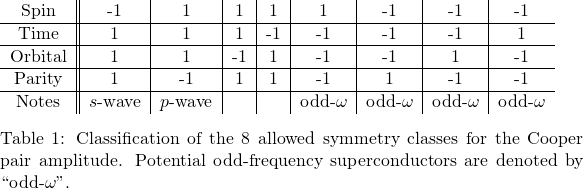
While no examples of bulk odd-frequency superconductors have yet been identified, there are a growing number of proposals for engineering these exotic amplitudes in heterostructures and driven systems, magnetic states and systems with Majorana modes.

Pair Symmetry Conversion in Driven Multiband Superconductors

By subjecting a multiband superconductor to a time-dependent drive, even-frequency pair amplitudes can be converted to odd-frequency pair amplitudes and vice versa. In the movie below we evaluate, as a function of time, both the even-frequency and odd-frequency pairing amplitudes of a multiband superconductor driven by a time-periodic chemical potential. At generic times during the period, contributions to the odd-frequency and even-frequency pair amplitudes are non-zero. The corrections to the odd-frequency amplitudes are largest exactly when the drive vanishes and smallest exactly when the drive reaches its maximum amplitude; the corrections to the even-ω amplitudes behave in the opposite manner.

Download video

Key papers:

Review article:
Odd-frequency superconductivity
Jacob Linder, Alexander V. Balatsky
arXiv:1709.03986
General conditions for proximity-induced odd-frequency superconductivity in two-dimensional electronic systems
Christopher Triola, Driss M. Badiane, Alexander V. Balatsky, E. Rossi
Phys. Rev. Lett. 116, 257001 (2016)
arXiv:1512.03068
Proximity-induced unconventional superconductivity in topological insulators
Annica M. Black-Schaffer, Alexander V. Balatsky
Phys. Rev. B 87, 220506(R) (2013)
arXiv:1305.4142

Nordita

Stockholm University

KTH

UConn